Page 183 - Chemistry ICSE Class IX

Study of Gas Laws 171

V
or = Constant (7.7)
T
where T is the temperature of the gas on the Kelvin scale.
Thus, the Charles’ law may also be stated as follows:
When the pressure is kept constant, the volume of a certain amount
of a gas varies directly with the absolute temperature of the gas.
Consider a certain amount of gas under constant pressure. Let
Volume of the gas = V 1
Temperature of the gas = T K
1
Then from the Charles’ law,
V
1 = Constant (7.8)
T 1
Now, if the temperature of the gas is changed to T (under constant
2
pressure), then
Volume of the gas = V 2
Temperature of the gas = T K
2
Then from the Charles’ law,
V
2 = Constant (7.9)
T 2

According to the Charles’ law
V V
1 = 2
T 1 T 2
Thus, if the same amount of gas is held at different temperatures, the
ratio of each volume-temperature (V – T) set would be constant, i.e.,
V V V
1 = 2 = 3 = ... ... = Constant
T 1 T 2 T 3

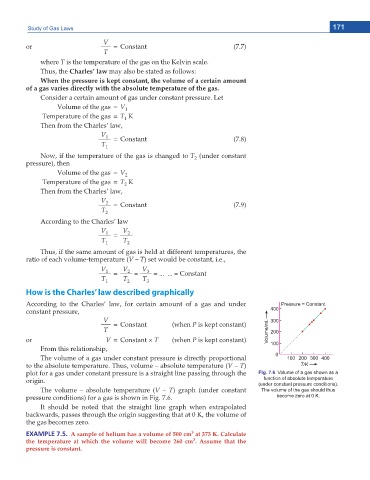
How is the Charles’ law described graphically
According to the Charles’ law, for certain amount of a gas and under
constant pressure,
V
= Constant (when P is kept constant)
T
or V = Constant × T (when P is kept constant)
From this relationship,
The volume of a gas under constant pressure is directly proportional
to the absolute temperature. Thus, volume – absolute temperature (V – T)
plot for a gas under constant pressure is a straight line passing through the Fig. 7.6 Volume of a gas shown as a
origin. function of absolute temperature
(under constant pressure conditions).
The volume – absolute temperature (V – T) graph (under constant The volume of the gas should thus
pressure conditions) for a gas is shown in Fig. 7.6. become zero at 0 K.
It should be noted that the straight line graph when extrapolated
backwards, passes through the origin suggesting that at 0 K, the volume of
the gas becomes zero.

3
EXAMPLE 7.5. A sample of helium has a volume of 500 cm at 373 K. Calculate
3
the temperature at which the volume will become 260 cm . Assume that the
pressure is constant.